All published articles of this journal are available on ScienceDirect.
Fuzzy Comprehensive Assessment Stability of Vegetated Slope with 3D Geomat Protection Using Cloud Model
Abstract
Based on the analysis of the classification criteria from various factors,a comprehensive evaluation system of the erosion stability of the vegetated eco-slope protected by 3D geomat is established. To solve the problem of uncertainty in the slope erosion stability analysis and consider the feature of both of random and fuzziness, the theory of Cloud Model has been applied into comprehensive evaluation to realize the uncertainty conversion between the qualitative concept and the quantitative data. By constructing the qualitative rule generator, the specific score of the evaluation index is determined according to the uncertain reasoning. The weights of the evaluation indexes are determined with the help of the Analytic Hierarchy Process developed by Cloud Model (CM-AHP), and then the overall score of the comprehensive evaluation system is obtained. The results of engineering examples demonstrate the practicability and effectiveness of the assessment model, which provides a new way for the evaluation of the erosion stability of the vegetated eco-slope protected by the 3D geomat.
1. BACKGROUND
In the construction of railway, highway, hydropower projects, the chances of slope excavation are always very high. The slope excavation may destroy the original vegetation and cause soil erosion, which may increase the degradation of the ecosystem. The traditional engineering slope protection technology, such as mortar or concrete protection, gunite, shotcrete and shotcrete-bolt slope protections, have made a great difference on slope protection. However, traditional slope protection technology are not environmentally friendly and the the cost of maintenance is very expensive. As a kind of composite ecological slope protection technology that combining 3D geomat and vegetation [1, 2], the vegetated eco-slope of 3D geomat protection technology is a combination of engineering and vegetation protection technology compared with the traditional slope protection technology. In the protection system, the 3D geomat is closely interwoven with the dense roots, thus making the root system integrated more uniformly and tightly, building a compactly complex system of the 3D geomat, the vegetation and the soil. The protection system works through the function of taproot anchoring, shallow root reinforcing, reducing pore water pressure of transpiration, intercepting rainfall and preventing slope runoff infiltration of vegetation, preventing the soil particles flowing through geomat. Besides, the honeycomb structure of 3D geomat can fix the planting soil on the slope,which provides essential soil and nutrients for the vegetation. The protection technology of vegetated eco-slope with 3D geomat has already been widely applied in the practical engineering because of the good effect for slope protection and ecological restoration.
However, there are some failure phenomena in practical engineering, such as the overall shear sliding along the slope of the protection system and partial erosion damage of the slope, making the ecological protection system ineffective. Especially at the initial formation stage of the protection system, rainfall may lead to poor stability of the protection system because the anti-rain-erosion function of the stems and leaves and the reinforcing and fastening soil function of root system cannot work, since the vegetation has not been restored yet. Once there is any erosion damage in the protection system, it will not only lead to the failure of the ecological restoration, but also make the original slope expose to the surrounding environment again, resulting in the potential dangers such as landslide, debris flow and other geological disasters. Therefore, it is of great theoretical and practical significance to analyze and evaluate the erosion stability of the vegetated eco-slope protected by 3D geomat safely, reliably and effectively.
In order to analyze and evaluate the uncertainty problems and probabilistic engineering mechanics problems in slope engineering effectively, various numerical simulation and system analysis methods are introduced to the slope stability research, such as the principle of limit equilibrium [3], Fuzzy Comprehensive Evaluation [4], Grey System Theory [5], Clustering Analysis Method [6], Expert System [7], Neural Network [8], Monte Carlo simulation [9] and Genetic Algorithm [10]. Each of these methods has its own characteristics and can reflect the complexity of the analysis and assessment of the stability of the slope to a degree, which has great importance to the construction and designing of the engineering of the vegetated eco-slope protected with 3D geomat. However, in practice the assessment of the erosion stability of the vegetated eco-slope depends very much on the engineering survey and the experience of the experts, which will inevitably lead to fuzziness and randomness in the process of the evaluation and the tradition research method often neglects. Therefore, the result of the evaluation still need to be improved.
Cloud Model, put forward by Academician Li Deyi from China [11], is a model of conversion between the qualitative and the quantitative, which is based on Fuzzy Mathematics theory and Probability Statistics. By endowing the sample point the randomness, fuzziness and relevance of the concept, the uncertainty conversion of the qualitative language and the quantitative expression is realized, which overcomes the shortcomings of the traditional evaluation method in neglecting of the fuzziness and randomness. In this paper, the Cloud Model is introduced into the comprehensive evaluation of the erosion stability. Firstly, according to Cloud Model Qualitative Rule Generator based on the Cloud Model theory, the score of the evaluation index is obtained. Then the weight of each index is determined according to the Analytic Hierarchy Process improved by Cloud Model (AHP-CM). And finally the evaluation result of the whole system is obtained by weighted multiplication. Engineering examples demonstrate the method has very important academic significance and engineering application value which enriches and perfects the theoretical system of the vegetated eco-slope protection with 3D geomat.
2. COMPREHENSIVE EVALUATION MODEL
2.1. Comprehensive Evaluation Index System
To evaluate the erosion stability of the vegetated eco-slope protected with 3D geomat, the influencing factors of the erosion stability should be determined at first. Many scholars have made theoretical analysis and experimental exploration to determine the influencing factors of the erosion stability. As mentioned above, the protection system is made up of 3 parts: the slope, the 3D geomat and the vegetation. Therefore, the parameters of the three parts have been used to assess the erosion stability of the protection system.
For the slope, according to Rough Set theory [12] and finite element analysis of ABAQUS [13],the main factors that affect the erosion stability are bulk density of soil, cohesion, friction angle, slope angle and ratio of pore pressure. Besides, slope length and compactness are also critical factors affecting erosion stability of artificial slope.
To the 3D geomat, the mechanism of slope protection of the 3D geomat is encapsulating the surface soil and preventing the soil particles flowing. Once there emerges tensile failure on the 3D geomat, the encapsulating function of protection system will be ineffective. Therefore, the main influencing factors are thickness and tensile strength of the 3D geomat [14]. Besides, based on Safety Factor Method [15], anchor spacing is another important factor affecting the design of similar engineering.
In the protection system, the main function of the vegetation are slope erosion prevention, reinforcement effect of roots and ecological restoration. Therefore, according to these functions, the main influencing factors are rate of vegetation coverage, roots development and planting suitability.
Precipitation condition is another important factor the erosion stability of the slope through the functions [3, 14] such as raindrop impact, runoff erosion, rainfall infiltration, especially at the initial establishment stage of the protection system. Therefore, the main influencing factors are rainfall intensity, rainfall duration and rainfall capacity.
Besides, some objective condition such as construction technology, maintenance method and environmental condition also affect the erosion stability of the slope. Especially, the factors such as temperature, humidity and PH value cannot be ignored for the vegetation restoration.
In a word, whether the evaluation indexes are too many or too few may lead to error of the evaluation. Based on previous research, seven categories of the factors are considered in this study: (a) characteristics of 3D geomat; (b) characteristics of soil; (c) characteristics of slope; (d) characteristics of vegetation; (e) characteristics of rainfall; (f) construction and maintenance; (g) environment. The factors and subfactors are as follows.
- (a). The characteristics of 3D geomat: (1) layer number of the geomat B11; (2) thickness of the geomat B12; (3) material of geomat B13.
- (b). The characteristics of soil: (1) cohesion of soil B21; (2) friction angle of soil B22; (3) bulk density of soil B23; (4) Permeability coefficient B24; (5) Degree of compaction B25; (6) pore pressure B26.
- (c). The characteristics of slope: (1) slope angel B31; (2) slope length B32; (3) lithology B33
- (d). The characteristics of vegetation: (1) vegetation coverage B41; (2) root development B42; (3) planting suitability B43.
- (e). The characteristics of rainfall: (1) rainfall intensity B51; (2) rainfall duration B52; (3) rainfall capacity B53.
- (f). Construction and maintenance: (1) construction technique B61; (2) maintenance way B62; (3) maintenance condition B63;
- (g). Environment: (1) temperature B71; (2) humidity B72; (3) PH value B73.
| Evaluation index | Grade | |||||
|---|---|---|---|---|---|---|
| grade I | gradeII | gradeIII | gradeIV | grade V | ||
| Characteristics of 3D geomat | Thickness of the geomat B11 (mm) | >16 | 14-16 | 12-14 | 10-12 | 8-10 |
| Tensile strength B12 (KN/m) | <1.0 | 1.0-1.5 | 1.5-2.0 | 2.0-2.5 | >2.5 | |
| Soil Nails Spacing B13 (m) | >3.2 | 2.2-3.2 | 1.5-2.2 | 0.8-1.2 | <0.8 | |
| The characteristics of soil | Cohesion of soil B21 (kPa) | >70.0 | 50.0-70.0 | 20.0-50.0 | 10.0-20.0 | <10.0 |
| Internal friction angle B22 (°) | >40.0 | 30.0-40.0 | 20.0-30.0 | 10.0-20.0 | <10.0 | |
| Bulk density B23 (KN/m3) | <12.0 | 12.0-16.0 | 16.0-20.0 | 20.0-24.0 | >24.0 | |
| Permeability coefficient B24 (cm/s) | 10-8-10-7 | 10-7-10-6 | 10-6-10-5 | 10-5-10-4 | 10-4-10-3 | |
| Compactness B25 (%) | 95-100 | 90-95 | 85-90 | 80-85 | 75-80 | |
| Ratio of pore pressure B26 | 0.45-0.5 | 0.4-0.45 | 0.35-0.4 | 0.3-0.35 | 0.25-0.3 | |
| The characteristics of slope | Slope angel B31 (°) | <25 | 25-45 | 45-65 | 65-75 | >75 |
| Slope length B32 (m) | >50 | 40-50 | 25-40 | 10-25 | <10 | |
| Lithology B33 | very good | good | moderate | bad | very bad | |
| The characteristics of vegetation | Vegetation coverage B41 (%) | >60 | 45-60 | 30-45 | 10-30 | <10 |
| Root development B42 | very good | good | moderate | bad | very bad | |
| Planting suitability B43 | very good | good | moderate | poor | very poor | |
| The characteristics of rainfall | Rainfall intensity B51 (mm/h) | <0.4 | 0.4-1.25 | 1.25-5.8 | 5.8-11.6 | >11.6 |
| Rainfall duration B52 (d) | <1.0 | 1.0-2.0 | 3.0-5.0 | 6.0-8.0 | >8.0 | |
| Daily rainfall capacity B53 (d) | <10.0 | 10.0-30.0 | 30.0-60.0 | 60.0-140 | >140.0 | |
| Construction and maintenance | Construction technique B61 | very good | good | moderate | bad | very bad |
| Maintenance condition B62 | very good | good | moderate | bad | very bad | |
| Maintenance time B63 (d) | >60 | 50-60 | 40-50 | 20-40 | <20 | |
| Environment | Temperature B71 (°C) | 25.0-30.0 | 20.0-25.0, | 10.0-20.0, | 0.0-10.0, | else |
| 30.0-35.0 | 35.0-40.0 | 40.0-45.0 | ||||
| Humidity B72(%) | >60 | 50-60 | 40-50 | 30-40 | <30 | |
| PH value B73 | else | 6.0-6.5, | 5.5-6.0, | 5.0-5.5, | else | |
| 7.5-8.0 | 8.0-8.5 | 8.5-9.0 | ||||
2.2. Comprehensive Evaluation Grade
The stability of slope is usually divided into five grades. Similarly, according to the stability of the slope, the erosion stability of the slope can also be divided into the following 5 grades: very stable (level I), stable (level II), basic stable (level III), unstable (level IV) and very unstable (level V). Besides, to maintain consistency with the evaluation grade, the evaluation index is divided into five grades, which is shown in the following Table 1.
2.3. Comprehensive Evaluation Model
Multiple-level comprehensive evaluation is a decision making technology that has been widely used for evaluation system with multiple factors and objectives. In this method, the evaluation index system is divided into a multiple-level structure, and the index weight of each layer is determined by AHP. The single index evaluation should be taken first and then the comprehensive evaluation is carried out to get the overall evaluation results by Calculating the single index evaluation result and its weight coefficient [16]. With the help of multilevel comprehensive evaluation, we can judge the erosion stability of the slope protected by the 3D geomat according to the following formula:
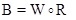 |
In the formula, W means weight coefficient of all the evaluation indexes. R means the single index evaluation result matrix. B means the final evaluation result matrix of comprehensive evaluation.
In fact, the comprehensive evaluation index system of erosion stability of vegetated slope protected with 3D geomat is complex and uncertain. The system not only contains the fuzziness, but also contains the uncertainty such as the randomness and the discreteness, which the multiple-level comprehensive evaluation does not take into consideration. Therefore, to evaluate the erosion stability more comprehensively and objectively, the Qualitative Rule Generator based on Cloud Model is introduced into the comprehensive evaluation.
3. COMPREHENSIVE EVALUATION BASED ON CLOUD MODEL
3.1. The Theory of Cloud Model [17]
Cloud model, proposed by Academician Li Deyi in 1990s, is uncertainty conversion model between the qualitative concept and the quantitative data, which mainly reflects uncertainty in the objective world and knowledge: fuzziness and randomness [12]. The cloud model integrates the two characteristics and constitutes the mutual mapping between the qualitative concept and quantitative data.
Based on the probability theory and fuzzy mathematics, Cloud Model expresses a concept with three numeral characteristic [18]: Ex (expected value), En(entropy) and He(hyper entropy), which combine the fuzziness, randomness and discreteness organically and realizes the uncertainty conversion between qualitative knowledge and quantitative data. Ex (expected value)is the most representative value of the qualitative concept and reflects the central position the cloud model; En (entropy), the uncertainty measure of the qualitative concept, reflects the degree of discreteness of cloud droplets which represent the randomness and fuzziness of qualitative concept; He(hyper entropy), determined by randomness and uncertainty of entropy, is an uncertainty measure of entropy, which reveals the relationship between the fuzziness and randomness.
Definition 3.1: Suppose U is a quantitative theory which is expressed by a certain value and C is a qualitative concept on U. If the quantitative value x is a random realization of the qualitative concept C and the certainty degree, μ (x) ,of x is a random number with stable tendency for C,which is to say:
 |
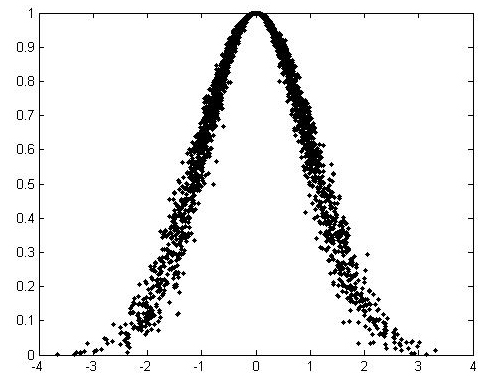
In this case, the distribution of X in the domain U is cloud. Each value of x is called a cloud droplet. Especially, if the number x satisfies x = RN (Ex, y ) (RN means Gaussian distribution) while y = RN (En, He ) and the certainty degree of x on U is
 , the cloud is called Normal Cloud Model. Normal Cloud Model is the most widely used cloud model and it has been proved to be generic [12]. The following picture show a cloud model that represents a qualitative concept (Fig. 1).
, the cloud is called Normal Cloud Model. Normal Cloud Model is the most widely used cloud model and it has been proved to be generic [12]. The following picture show a cloud model that represents a qualitative concept (Fig. 1).
3.2. Qualitative Rule Generator of Cloud Model [18]
Definition 3.2: Forward Normal Cloud Generator (FCG) is a mapping from the qualitative concept to the quantitative data, which generates the cloud droplets according to the numerical characteristics (Ex, En, He).
Definition 3.3: Front Member Cloud Generator (FMCG) refers to the Forward Cloud Generator that generates membership degree distribution of a given point according to the point and numerical characteristics of cloud model.
Definition 3.4: Behind Member Cloud Generator (BMCG) refers to the Forward Cloud Generator that generates cloud droplet distribution according to the given membership degree and numerical characteristics of cloud model.
Definition 3.5: Backward Normal Cloud Generator (BCG) is a mapping from the quantitative to the qualitative, which converts a certain number of accurate data into a qualitative concept represented by numerical characteristics (Ex, En, He).
Definition 3.6: Qualitative Rule Generator (QRG), important application of Cloud Model theory, is consisted of Front Member Cloud Generator and Behind Member Cloud Generator to realize the uncertain conversion between the qualitative knowledge and quantitative data.
Qualitative Rule Generator based on Cloud Model may have multiple rules and multiple conditions. For the evaluation system of erosion stability, the article chooses the Qualitative Rule Generator with single condition and single rule. Its conceptual diagram and algorithm is shown in the following part (Fig. 2).
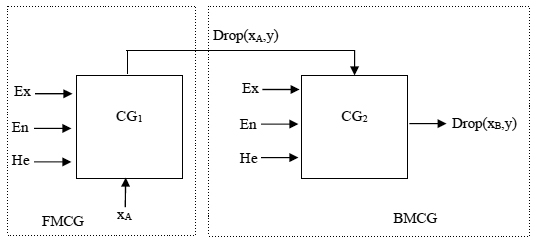
Algorithm 3.1
Input: Three numeral characteristics (ExA, EnA HeA ) of front member A and the quantitative value xA, three numeral characteristics (ExB, EnB HeB ) of behind member B.
Output: cloud droplet xB of behind member and its membership grade μ.
(1) Generate a random number En'A that meets Rn( En A, He A );
(2) Calculate the membership grade μ:
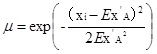 |
(1) |
(3) Generate a random number En'B that meets Rn( EnB, HeB );
(4) If the inputted value activates the rising phase of the front member, the behind member choose the rising phase: If xA ≤ ExA, then
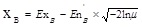 ;
;
(5) If the inputted value activates the decline phase of the front member, the behind member choose the decline phase: If xA > ExA, then
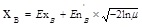 ;
;
After the Cloud Model theory is applied into the erosion stability evaluation system, this study will take the uncertainty such as fuzziness and randomness of the evaluation system into consideration, making the result of the evaluation more objective and accurate.
3.3. Improved AHP with Cloud Model [19]
Analytic Hierarchy Process(AHP), put forward by American operation research scientist Satty.T.L [20], is a multiple criteria decision analysis method. It is flexible and can combine the qualitative and quantitative analysis. The traditional AHP use classical Satty scale (1-9) to describe the relative importance of different factors, and the weight of each index is calculated by constructing the comparison judgment matrix. However, using accurate number to express the relative importance may ignore the uncertainty of the relative importance. Besides, there may has randomness and fuzziness in the process of determining the relative importance value by the experts. Considering these factors, this study introduces the Cloud Model into the AHP by using the Backward Normal Cloud Generator to calculate the relative importance and optimize the the result of weight calculation .
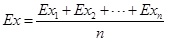 |
(2) |
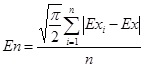 |
(3) |
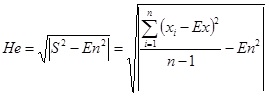 |
(4) |
(1) Invite N experts to judge the relative importance of the index. With the help of the Backward Normal Cloud Generator, we can generate a decision cloud about the judgment of experts.
(2) Build the judgment matrix of relative importance based on cloud model. First, the judgment matrix of relative importance is obtained according to group decision-making:
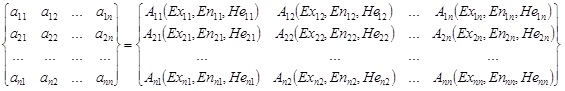 |
On the diagonal, the hyper entropy and the entropy of the cloud model is 0 and the expected value is 1. Aij is the reciprocal of Aji, and according to the reciprocal algorithm of cloud model they satisfy the following formula:
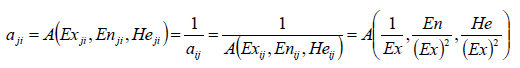 |
(5) |
(3) Calculate the relative weight coefficient of the evaluation elements according to root method:
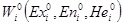 . According to the multiplication of the cloud model, the calculation results of
. According to the multiplication of the cloud model, the calculation results of
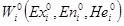 are listed as the following:
are listed as the following:
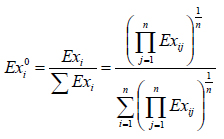 |
(6) |
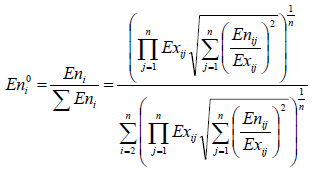 |
(7) |
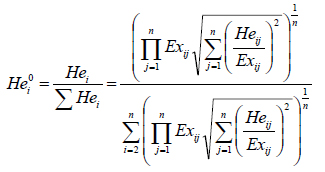 |
(8) |
(4) Take consistency test. The consistency test method for the three numerical characteristics of cloud model is consistent. Usually, we only take consistency test for the numerical characteristic Ex. The index of conformance test C.I. is
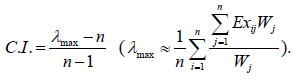 |
The index of consistency text is consistent with the classical AHP. C.R.,the proportion of conformance test, satisfies the following formula: C.R. < 0.1.
3.4. The Procedure of Comprehensive Evaluation
(1) Classify the indexes of the comprehensive evaluation system according to the classification of comment set. Normalize [21] the variables with different dimensionless and predetermine the range of variation for the different grades.
For the influencing factor that the bigger the better, data normalization can be implemented as:
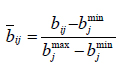 |
(9) |
For the influencing factor that the smaller the better, data normalization can be implemented as:
 |
(10) |
(2) Format the cloud model of the comments set. According to the range of the comments set and the definition of the score of comments set, the three numerical characteristics of the cloud model are determined [22].
a) As mentioned above, EX is the expected value of sample points that meet normal distribution, therefore,
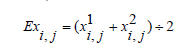 |
(11) |
In the formula, Exi, j means the expected value of factor ‘i’ of grade ‘j’. x1i, j means the maximum value and x2i, j means minimum value in the section of different grades.
b) In order to show the boundary fuzziness of adjacent grades, the membership of the two adjacent grades is equal. Therefore, the En (entropy)is determined by the following equation:
 |
(12) |
 |
(13) |
c) The He(hyper entropy)is usually determined by experience. The value of He(hyper entropy) is usually less than 0.05. The paper make the value as He = 0.01.
(3) Evaluate the single factor of the evaluation system by qualitative rules generator based on cloud model according to the uncertain reasoning method.
(4) Determine the weight coefficient of the index of the evaluation system. The article selects AHP improved with Cloud Model, considering the fuzziness and randomness of the subjective factors in the evaluation process.
(5) Calculate the score of each evaluation index with its weight coefficient, then we can obtain the final score of the comprehensive evaluation, further we can obtain the evaluation grade of the erosion stability.
4. THE ANALYSIS OF ENGINEERING CASE
Qinghong expressway is located in the east Shandong Province. The speed limit of the expressway is 120 km/h and the width of the roadbed is 34.5 m. The design slope angel is 40°and the design length of the slope is 15m. Geomat protection of slope has been used to protect the erosion stability of the slope. To evaluate the erosion stability, the comprehensive evaluation based on Cloud Model is applied and the Table 2 shows the relative data of Qinghong Expressway and its normalization.
| Evaluation factor | Original | Normalization | Evaluation factor | Original | Normalization |
|---|---|---|---|---|---|
| Thickness (mm) Tensile strength (KN/m) Soil Nails Spacing (m) Cohesion of soil ( kPa ) Internal friction angle (°) Bulk density (KN/m3) Permeability coefficient (cm/s) Compactness (%) Ratio of pore pressure Slope angel (°) Slope length (m) Lithology |
14 mm | 0.75 | Vegetation coverage (%) Root development Planting suitability Rainfall intensity (mm/h) Rainfall duration (d) Daily rainfall capacity (mm) Construction technique Maintenance condition Maintenance time (d) Temperature (°C) Humidity(%) PH value |
40% | 0.59 |
| 2 KN/m | 0.5 | good | 0.7 | ||
| 1 m | 0.95 | good | 0.7 | ||
| 62.92kPa | 0.899 | 2.5 mm/h | 0.78 | ||
| 23.09° | 0.577 | 0.5 day | 0.938 | ||
| 18.7 KN/m3 | 0.221 | 5 mm | 0.964 | ||
| 3.2×10-6cm/s | 0.72 | good | 0.7 | ||
| 90% | 0.8 | moderate | 0.5 | ||
| 0.3 | 0.2 | 45 day | 0.5 | ||
| 40° | 0.66 | 16°C | 0.533 | ||
| 15 m | 0.17 | 50% | 0.833 | ||
| poor | 0.3 | 8.4 | 0.35 |
| Evaluation index | Grade | |||||
|---|---|---|---|---|---|---|
| grade I | gradeII | gradeIII | gradeIV | grade V | ||
| characteristics of 3D geomat | Thickness of the geomat B11 (mm) Tensile strength B12 (KN/m) Soil Nails Spacing B13 (m) |
0.95-1 0.95-1 0.95-1 |
0.75-0.95 0.65-0.95 0.65-0.95 |
0.5-0.75 0.35-0.65 0.35-0.65 |
0.25-0.5 0.05-0.35 0.05-0.35 |
0-0.25 0-0.05 0-0.05 |
| The characteristics of soil | Cohesion of soil B21 ( kPa ) Internal friction angle B22 (°) Bulk density B23 (KN/m3) Permeability coefficient B24 (cm/s) Compactness B25 (%) Ratio of pore pressure B26 |
0.875-1 0.8-1 0.95-1 0.95-1 0.8-1 0.8-1 |
0.625-0.875 0.6-0.8 0.65-0.95 0.65-0.95 0.6-0.8 0.6-0.8 |
0.25-0.625 0.4-0.6 0.35-0.65 0.35-0.65 0.4-0.6 0.4-0.6 |
0.125-0.25 0.2-0.4 0.05-0.35 0.05-0.35 0.2-0.4 0.2-0.4 |
0-0.125 0-0.2 0-0.05 0-0.05 0-0.2 0-0.2 |
| The characteristics of slope | Slope angel B31 (°) Slope length B32 (m) Lithology B33 |
0.9-1 0.9-1 0.8-1 |
0.58-0.9 0.7-0.9 0.6-0.8 |
0.26-0.58 0.4-0.7 0.4-0.6 |
0.1-0.26 0.1-0.4 0.2-0.4 |
0-0.1 0-0.1 0-0.2 |
| The characteristics of vegetation | Vegetation coverage B41 (%) Root development B42 Planting suitability B43 |
0.95-1 0.8-1 0.8-1 |
0.65-0.95 0.6-0.8 0.6-0.8 |
0.35-0.65 0.4-0.6 0.4-0.6 |
0.05-0.35 0.2-0.4 0.2-0.4 |
0-0.05 0-0.2 0-0.2 |
| The characteristics of rainfall | Rainfall intensity B51 (mm/h) Rainfall duration B52 (d) Daily rainfall capacity B53 (d) |
0.95-1 0.95-1 0.95-1 |
0.9-0.95 0.82-0.95 0.81-0.0.95 |
0.5-0.9 0.44-0.82 0.6-0.81 |
0.05-0.5 0.05-0.44 0.05--0.6 |
0-0.05 0-0.05 0-0.05 |
| Construction and maintenance | Construction technique B61 Maintenance condition B62 Maintenance time B63 (d) |
0.8-1 0.8-1 0.95-1 |
0.6-0.8 0.6-0.8 0.65-0.95 |
0.4-0.6 0.4-0.6 0.35-0.65 |
0.2-0.4 0.2-0.4 0.05-0.35 |
0-0.2 0-0.2 0-0.05 |
| Environment | Temperature B71 (°C) Humidity B72(%) PH value B73 |
0.8-1 0.95-1 0.8-1 |
0.6-0.8 0.65-0.95 0.6-0.8 |
0.4-0.6 0.35-0.65 0.4-0.6 |
0.2-0.4 0.05-0.35 0.2-0.4 |
0-0.2 0-0.05 0-0.2 |
4.1. The Classification and the Normalization of the Evaluation Index
In this paper, the erosion stability of the slope protected by 3D geomat is divided into 5 grades: Very stable (grade I), stable (gradeII), basically stable (gradeIII), unstable (gradeIV), very unstable (grade V). In order to keep the consistency of the forward cloud model and backward cloud model, classify the evaluation index into 5 grades and normalize the date of the evaluation index. The result of the classification and normalization is given in the Table 3.
4.2. Build the Front Member Cloud Model and Behind Member Cloud Model
According to the variation range of the classification for each index and the formula (3-9) ~ (3-12), the front member cloud model is developed. For example, for the index ‘the thickness of 3D geomat’, the cloud model (Ex,En,He) are: (0.975, 0.05/2.355, 0.01), (0.8, 0.3/2.355, 0.01), (0.5, 0.3/2.355, 0.01), (0.2, 0.3/2.355, 0.01), (0.025, 0.05/2.355, 0.01) corresponding to its qualitative comment ‘very stable, stable, generally stable, unstable and very unstable’.
For the behind member cloud model, assuming that the better the index is, the higher the score is. If the full mark is 100, the cloud model are: (90, 20/2.355, 0.01), (70, 20/2.355, 0.01), (50, 20/2.355, 0.01), (30, 20/2.355, 0.01), (10, 20/2.355, 0.01) [23] corresponding to its qualitative comment ‘very high, high, medium, low and very low’.
4.3. Determine Weight Coefficient
The study uses AHP developed with Cloud Model (CM-AHP) to calculate the weight coefficient of the evaluation index to make the calculation results more objective. The calculation procedure is shown in the chapter 3.3. The article take the index B1 “the characteristics of the 3D geomat “ as an example to show the calculation procedure, the result is given in the Table 4. And the calculation results of the weights of all indexes are given in the Table 5.
| B | B11 B12 B13 |
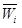
|
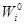
|
|---|---|---|---|
| B11 B11 B13 |
(1,0,0) (4, 0.836, 0.050) (0.260, 0.054, 0.011) (0.25, 0.053, 0.003) (1, 0, 0) (0.145, 0.018, 0.003) (3.846, 0.799, 0.163) (6.897, 0.856, 0.143) (1,0,0) |
(1.013,0.674, 0.358) (0.331, 0.207, 0.095) (2.982, 1.858,1.078) |
(0.234, 0.246, 0.234) (0.077, 0.076, 0.062) (0.689, 0.678, 0.704) |
| Score | 1st index | Weight | Score | 2nd index | Weight | Score |
|---|---|---|---|---|---|---|
| 58.921 | characteristics of 3D geomat | 0.217 | 72.760 | Soil Nails Spacing (m) Thickness of the geomat (mm) Tensile strength of geomat (KN/m) |
0.234 0.077 0.689 |
60.358 50.000 79.515 |
| The characteristics of soil | 0.147 | 47.953 | Cohesion of soil ( kPa ) Internal friction angle (°) Bulk density (KN/m3) Permeability coefficient (cm/s) Compactness (%) Ratio of pore pressure |
0.100 0.039 0.458 0.312 0.056 0.035 |
62.805 46.567 31.585 64.472 80.875 21.318 |
|
| The characteristics of slope | 0.353 | 48.653 | Slope angel (°) Slope length (m) Lithology |
0.578 0.309 0.113 |
65.413 24.125 30.000 |
|
| The characteristics of vegetation | 0.138 | 64.332 | Vegetation coverage (%) Root development Planting suitability |
0.392 0.406 0.202 |
55.410 70.000 70.254 |
|
| The characteristics of rainfall | 0.078 | 77.100 | Rainfall intensity (mm/h) Rainfall duration (d) Daily rainfall capacity (d) |
0.582 0.256 0.162 |
73.877 78.882 85.862 |
|
| Construction and maintenance | 0.048 | 61.440 | Construction technique Maintenance condition Maintenance time (d) |
0.572 0.286 0.142 |
70.000 50.000 50.000 |
|
| Environment | 0.019 | 56.177 | Temperature (°C) Humidity(%) PH value |
0.324 0.126 0.550 |
51.198 71.198 55.669 |
4.4. Single Factor Evaluation
According to the uncertainty reasoning based on cloud model, the qualitative language description of evaluation index is quantified as the certain score with the help of Qualitative Rule Generator based on cloud model. The calculation results are provided in the Table 5.
4.5. The Comprehensive Evaluation
Multiply the score of the evaluation index with the weight coefficient to get comprehensive evaluation score. The score of comprehensive evaluation based on cloud model is 58.921 and the grade of the erosion stability is grade III. To prove the feasibility of the cloud model in comprehensive evaluation, we use the fuzzy matter-element [24] to evaluate the same example. The result shows consistent with the result of the comprehensive evaluation using cloud model.
CONCLUSION
With the increasing number of slope engineering, the traditional slope protection technology is no longer able to meet the requirements no matter on economics or environment. As a kind of new technology, ecological slope protection technology is beneficial to environmental protection. It will not only protect slope stability and prevent erosion, but also will combine the slope engineering with the surrounding ecological landscape which is environmentally friendly. The analysis of erosion stability of vegetated eco-slope protected by 3D geomat is the basic work of the design of the engineering. There are many factors that affect the erosion stability of slope. Besides, the randomness and fuzziness of these factors increase the uncertainty of the evaluation. In this paper, the Cloud Model is applied into the comprehensive evaluation of the erosion stability of vegetated eco-slope protected by 3D geomat. With the help of MATLAB [25] program, the article builds a qualitative rule generator based on cloud model to realize the conversion between the qualitative concept and the quantitative data. Through the analysis of engineering case, the evaluation method is proved to be feasible and easy to be calculated, which provides a new method for the analysis of the erosion stability of the vegetated slope by 3D geomat. Compared with the traditional evaluation method, on one hand the new method will no longer consider membership functions and on the other hand it considers the fuzziness and randomness of the evaluation process fully by introducing the Cloud Model. The Cloud Model optimize the theoretical system of the research on the erosion stability of the vegetated eco-slope protected by the 3D geomat and has important guiding significance to practical engineering.
CONFLICT OF INTEREST
The authors confirm that this article content has no conflict of interest.
ACKNOWLEDGEMENTS
The supports given to this work by the National Natural Science Foundation of China (No. 11372165) are greatly acknowledged.


